Homework 10

Due: April 3, 2018, 8 a.m.

Please give a complete, justified solution to each question below. A single-term answer without explanation will receive no credit.

Please complete each question on its own sheet of paper (or more if necessary), and upload to Gradsescope.

$$ \newcommand{\R}{\mathbb{R}} \newcommand{\dydx}{\frac{dy}{dx}} \newcommand{\proj}{\textrm{proj}} % For boldface vectors: \renewcommand{\vec}[1]{\mathbf{#1}} $$

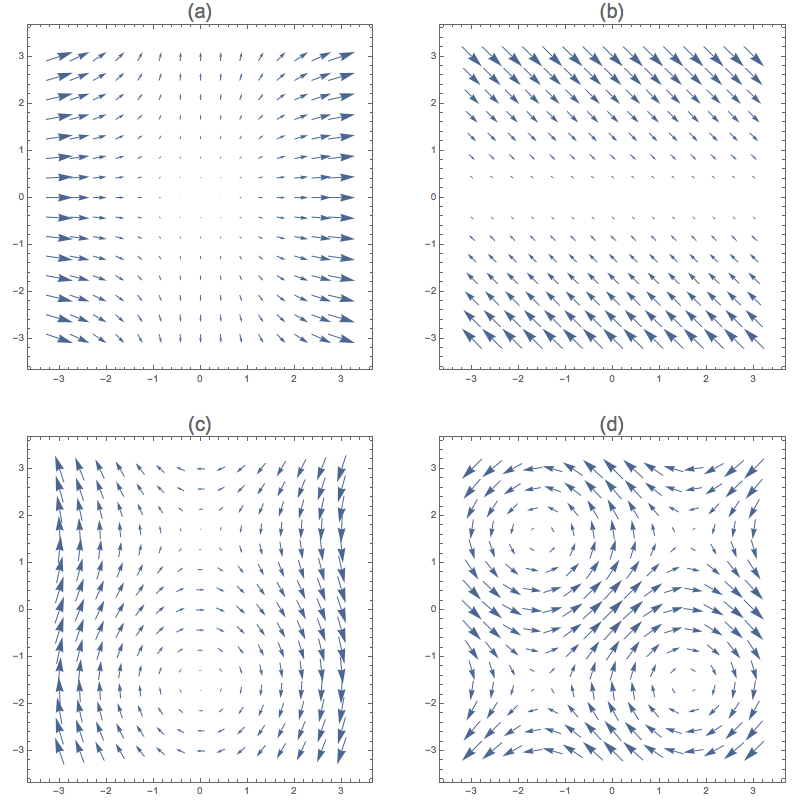
1. Each vctor field below has the form $\vec F(x,y) = P(x,y)\,\vec i + Q(x,y)\,\vec j$ where $P$ and $Q$ are sepelcted from among the functions $$\{\pm x,\pm y,\pm x^2,\pm y^2,\pm \cos(x),\pm \cos y \}.$$

For each, find $P$ and $Q$ and briefly describe how you arrived at your conclusion.

2. Determine a potential function $f(x,y,z)$ for the following conservative vector field.

$$ \vec F(x,y,z) = \left(\frac{1}{x}+y z^2-3\right)\,\vec i+\left(x z^2-z \sin (y z)\right)\,\vec j +\left(2 x y z-y \sin (y z)+\frac{1}{z}\right)\,\vec k $$

3. Compute the line integral $ \int_C f \,ds$ (i.e., with respect to arclength) where the curve $C$ is graphed below (oriented counterclockwise) and $$f(x,y) = x^2 + y^2. $$

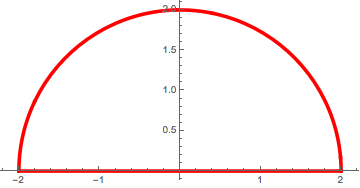
(You may use the interpretation $\int_C f\,ds$ given in class and/or use the formula $$\int_c f\,ds = \int_a^b f(\vec r(t)) |\vec r'(t)|\,dt $$ as explained in §16.2 of the text.)