Homework 9

Due: March 27, 2018, 8 a.m.

Please give a complete, justified solution to each question below. A single-term answer without explanation will receive no credit.

Please complete each question on its own sheet of paper (or more if necessary), and upload to Gradsescope.

$$ \newcommand{\R}{\mathbb{R}} \newcommand{\dydx}{\frac{dy}{dx}} \newcommand{\proj}{\textrm{proj}} % For boldface vectors: \renewcommand{\vec}[1]{\mathbf{#1}} $$

1. Sketch the region of integration of the of the following iterated integral and then re-express it in "$dx\,dz\,dy$" order.

$$ \int_{-1}^0\int_{-\sqrt{1-x^2}}^{\sqrt{1-x^2}} \int_0^{y+2} f(x,y,z) \,dz\, dy\, dx $$ Finally, reexpress it in cylindrical coordinates.

2. Find the volume of the following "stadium" using spherical coordinates. The "inner" wall is a piece of a sphere of radius 2; the "stands" are part of the cone $z^2=x^2+y^2$.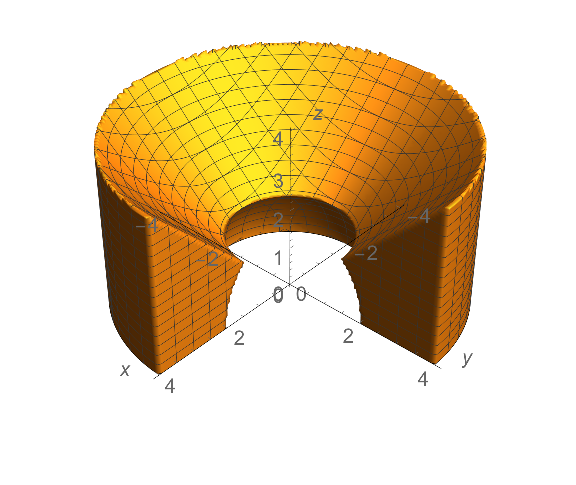

3. Find the center of mass $(\overline{x},\overline{y},\overline{z})$ of the "ice cream cone" from homework 8 (that is the region above the cone $z=\sqrt{x^2+y^2}$ and inside the sqphere $x^2+y^2+z^2=8$). Assume the ice cream has uniform density.

Hint: You may reason your way to 2 of the coordinates without computing anything directly, but you must set up and evaluate an integral (or integrals) for the third.

4. Repeat question 3 but assume the ice cream in the cone (i.e., that below the rim) is twice as dense as that above.